Scribe: (DABC) Sine/Cosine; Relations and Modes of Representation

As your scribe for today, I will put my efforts into defining today's lesson. I hope that standards will be met and that this may be used to a greater extent as a future reference.

Today, we learned more about trig functions. We learned how to sketch the graphs, interpret the information, and undo our steps to either find equations from analyzing the graph or vice versa. There were many things that we went over today, upon which I will try to re-explain.

Class 1: Morning

To start the class off, we put our thoughts into questions that were written on the board.

1.) On the same cartesian plane, using 2 different colours, sketch at least 2 cycles of:

f(x) = Cos(x) and g(x) = Sin(x)



As Mr. K stated during the class, graphing these functions are as easy as counting from 1-4. In this example, upon which the graph is in intervals of Π/2, you would label your scale as Π/2, Π, 3Π/2, and 2Π on the positive side of the x-axis. On the negative side of the x-axis, you would label the scale with the same values except that they are negative: -Π/2, -Π, -3Π/2, and -2Π.

There are things to remember when graphing in order to achieve full marks. Some of which are quite simple:

- label your axis
- add arrows to your axis and your curves
- **make certain that the curve arrows either point up or down, NOT STRAIGHT

Back to the question, there are things that you should notice about the function of Sin(x) and Cos(x).

- both "wrap" around a line, known as the "sinusoidal axis" or the "average value of the function"
- Cosine starts at its max value
- Sine starts at its sinusoidal axis

Exploring further into the concept, we find that we can rewrite the function of Sin(x) in terms of Cosine, and rewrite the function of Cos(x) in terms of Sine.

Sin in terms of Cos:
-> Cos(x- Π/2) = Sin(x)

Cos in terms of Sin:
-> Sin(x+ Π/2) = Cos(x)


NOTE: Π/2 in both of these equations are the phase shift a long the horizontal axis, either left or right, depending on its sign, which will be discussed further on in this post.

2.) Without using a calculator, sketch each of the following graphs:


a.) y = Sin(x) - 1


b.) y = -2Sin(x)

Notice that the amplitude, in this case (2) is not negative because amplitudes are described as distances and therefore should not be negative.


c.) y = 2Sin(x) + 1


In graphing these functions, there are certain steps that may be followed to help make graphing easier also explained later in this post.

- - - - - - - - - -

-In general or standard form, the equation of sine functions are:

• f(x) = ASinB(x-C) + D

-Cosine is affected by the same transformations:

• f(x) = ACosB(x-C) + D

A- The value of A relates both to the amplitude and whether the function will be inverted or not over the y-axis. The amplitude (which is the absolute value of A: |A|) of the graph is the distance from the sinusoidal axis. Its sign influences whether the curve will "flip" over the y-axis or if it retains its normal position. Further explained later on.

B- Parameter B is not the period of the graph but helps determine the period. This is also explained further into the class.

C- The phase shift/ horizontal shift of the graph.

D- Parameter D is the sinusoidal axis, average value of the function, or the vertical shift.

- - - - - - - - - -

Now, having discovered the properties of the transformations, we dive deeper into the concept and talk about how these variables effect the graph and how graphing can be put into an easier form of remembering.

Ex. Sin2x

• the value of "B" causes "everything to happen twice as fast"

• the angle is "doubled" before finding the value of sine

Ex. Sin-2x

• Inputs are made negative first

• Note: x-coordinates are changing sign

• Note: the negative signs do not flip the graph vertically over the x-axis but horizontally over the y-axis

**To help us improve our understanding of these examples, we compared them with Sin(x) on the same Cartesian plane.

Now diving even deeper with this new knowledge, we compare more examples to notice occurring patterns (mathematics is the study of patterns). We do this by observing sketches of functions.

Sinx = 1/2 <-- 1 wave between 0-2Π
Sin2x = 1/2 <-- 2 waves between 0-2Π
Sin3x = 1/2 <-- 3 waves between 0-2Π Sin4x = 1/2 <--4 waves between 0-2Π So, we find that the value of "B" is somehow related to the number of waves between a given interval (in this case 0-2Π). However, when the word "Period" comes to mind, people ask themselves, is the value of "B" in fact the period? The answer to that is no. "B" is in fact not the period of the graph, but indeed helps determine it.

• A period is the distance of a hill and a wave.

Using this mode of representation, we can find the relationship between the value of "B" and the period.

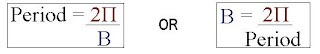Now discovering all of this in the first class of the day may be quite a load. However, one thing that should definitely not be forgotten is the concept of the mnemonic: DABC. At first, the abbreviation may look unfamiliar. However when you peer closer, you notice that A, B, C, and D, are part of the transformational equation of Sine and Cosine! DABC is actually that, except in the form of order upon which can be helpful in remembering how to sketch trig functions.

D - Is the first step in sketching the graph of a trig function. D is the vertical shift or sinusoidal axis of the graph and should be found first, as you should know that the graph "wraps" around the sinusoidal axis.

A - Is the second step in sketching the graph of a trig function. A is the amplitude and determines the stretch of the graph. Also important about the amplitude is its sign. If it is negative, the graph appears to be inverted; it flips horizontally over the y-axis. If positive, Sine graphs will start at zero, and Cosine graphs will start at its max value, which is one.

B - Is the third step in sketching the graph of a trig function. B represents a factor that influences the period of the graph. This is used to determine the scale values of the x-axis.

C - Last but not least, the last step in sketching the graph of a trig function. C represents the horizontal shift of the graph.

When thinking of graphing one of these monsters, you may curse in the form of DABC (pronounced: Dah - Bick!). Then all of a sudden, a flash of insight washes over you and you suddenly remember how to sketch the graph! Isn't that amazing everyone?

Finally, we reached the last couple minutes of a long class (or short?). Mr. K put up an example, which he ended up sketching quickly on the board:

y= -3Cos2(x - Π/4) + 1



For indication purposes, during the class, Mr. K compared graphing trig functions to the "etchisketch" which is quite the analogy. He stated that one dial of the etchisketch could be compared to Sin and the other dial could be compared to Cos. Imagine both of the dials being turned simultaneously and the result is quite frankly circular functions displayed in a graphical manner or atleast visual.

Class 2: Afternoon

We started off the afternoon by taking a look at these two previous equations:

f(x) = AsinB(x-C)+D
f(x) = AcosB(x-C)+D

Discussing briefly about the two, we move quickly to an example.

Ex. y = -2sin3(x+Π/6)+1

• Note: The vertical shift is to the left in this example. The positive sign is due to a negative value input replacing the variable C. Since the formula has a negative sign, and the example has a positive sign, the horizontal shift is to the left because a (-)(-) = (+).
• Note: When solving this equation, for any reason, simply use the order of operations: BEDMAS

Now having graphed this function, Mr. K talked brought up a great topic. He said that, if you know how to do something one way in math, you should know how to undo it. So, that's what we ended up doing. Instead of converting the equation to a graphical mode of representation, we reversed the method and converted the graphs into symbolic modes of representation: in the form of an equation.

So you might ask, how do you do that? Well, we started off by find the values ABCD of the transformational equations of sine or cosine.

Note: For this one graph, there can be a large (when I say large, I mean LARGE) quantity of equations that when graphed, will all look similar or are exact replicates.

We decided to rewrite the equation in Cosine:

• A= -2

• B= 3 (B= 2Π/p = 2Π/2Π/3 = 3)

• C= 0 (normal cosine curves start at its max, whiel this graph starts at its min due to the negative amplitude sign)

• D= 1

By substituting the numbers back into the framework equation, we get:

y=-2cos3x+1

Next we decided to rewrite the equation 3 more times: 2 in terms of cosine, and 1 in terms of sine.

Sine

• A= 2
• B= 3
• C= Π/6
• D= 1

y=2sin3(x-Π/6) + 1

Cosine1

• A= 2
• B= 3
• C= -Π/3
• D= 1

y=2cos3(x+Π/3) + 1

Cosine2

• A= 2
• B= 3
• C= -Π/3
• D= 1

y=2cos3(x+Π/3) + 1

After all those nice examples, we look at where the quadrants are located in the graph. To put it short, depending on where your starting point is, the period of the curve is divided by 4 pieces, quadrant 1, 2, 3, and 4 before repeating itself again.

After this, we quickly went over number 15 of exercise #5, and number 12 of exercise #6.

We are now DONEEEEEEEEEEEEEEEEEEEEEEEEEEEEEEEEEEEE the unit and our pretest is on its way (supposedly thursday). Tomorrow, we are taking notes into our super duper "Math Dictionary of Power" (lol) and are going over any issues, concerns, and questions about the unit! Make sure to ask some questions or we'll just have wasted a class that could've been used towards our exam studying time near the end.

WOW THAT WAS A DOOZY. Now we near the end.

Any comments, suggestions, error fixing, criticism, appreciation, is accepted :)

Homework for tonight: Curve sketching posted by Mr. K (so far has not appeared)

Scribe for tomorrow: Bertman! (sorry Sam, Bert asked me first :P)

Have a nice day. Night.